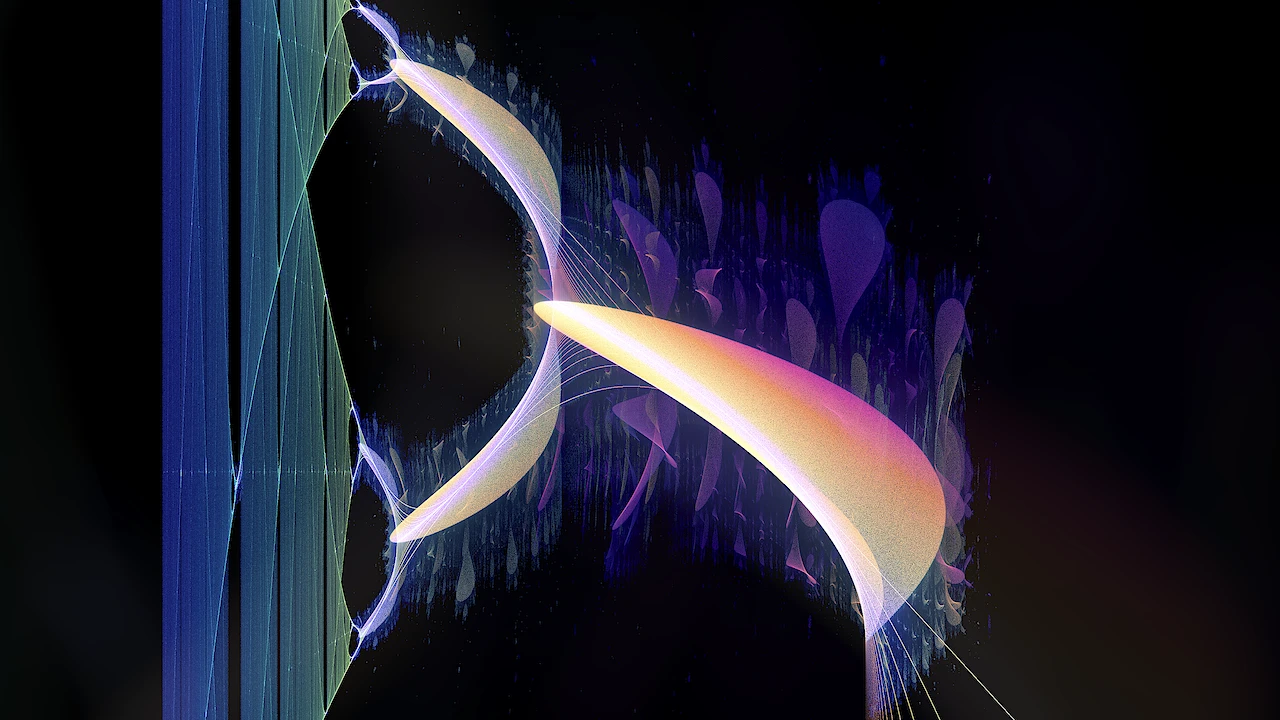
This composite image reveals the bifurcation diagram embedded within the Buddhabrot fractal. It is essentially an infinitely thin Buddhabrot cross section which straddles the real-axis. Since there is no imaginary component in any of the c values chosen the resulting zₙ sequences have none either and as a result the whole structure of the bifurcation diagram is constrained to the ZrCr plane. The z₁=c diagonal which acts as the upper bound of the bifurcation diagram can be seen going from bottom right to the top left. The parabolic z₂=c²+c curve acts as a lower bound, but only for the negative c points.

The dark "absorption" gaps on the chaotic left-side of the diagram are regions of intermittent stability where smaller Buddhabrot copies of varying periodicities can be found. To the right the behavior of the curve is purely exponential so it simply converges into a vertical line as we iterate it further (not bound by the parabolic z₂). The point where that vertical line and the main cardioid of the stable Anti-Buddhabrot meet is c=¼ – the termination point of the 'Elephant valley'.

To get a better sense of what the bifurcation pattern embedded in the Anti-Buddhabrot looks like on its own you can browse the other related images here.