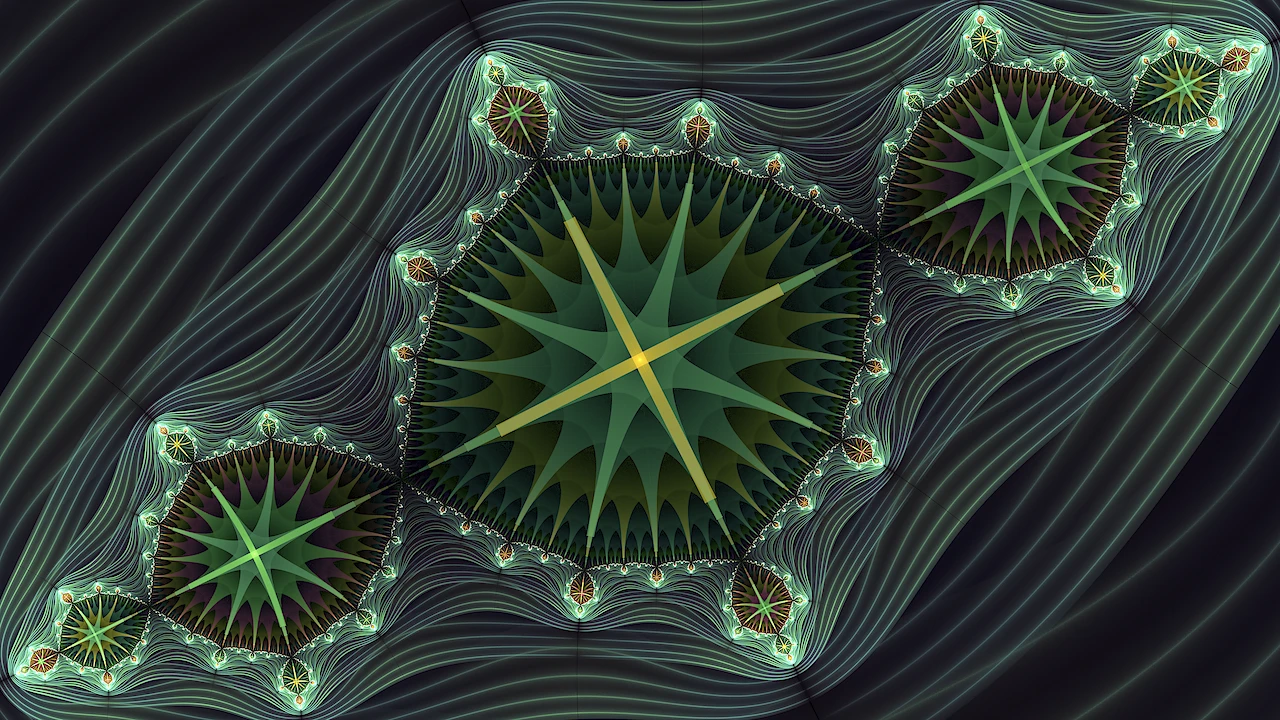
A Mandelroot rendering of the period-2 locus at c=-1. The boundary that separates the internal and external dynamics is the same that can be seen in some of my Julia set renderings. While the outer region seems to more or less uniformly converge on the boundary as the iterations advance, the inner structure appears much more complicated. It carries a certain resemblance to some of the hyperbolic works of M.C. Escher (see 'Circle Limit III' for what I mean).

Escher did his work by hand, but here the internal structure is of course computer-generated. First, we start out with a cross-shape (highlighted in yellow in the middle). Then, all the points inside that shape are fed through an iterative function which simply swaps the z²-term of the standard Mandelbrot for a square root of z which explains the name Mandelroot. Since a square root always has two answers the total number of rendered crosses doubles for each iteration, and after only forty iterations there are a total of over one trillion crosses (most much smaller than a pixel). Still, each one of them is just a translated, rotated, scaled, and slightly deformed copy of the original one. When all iteration steps are displayed at once the internal hyperbolic geometry of the Julia bulbs is revealed.

This video shows the larger context for this image.