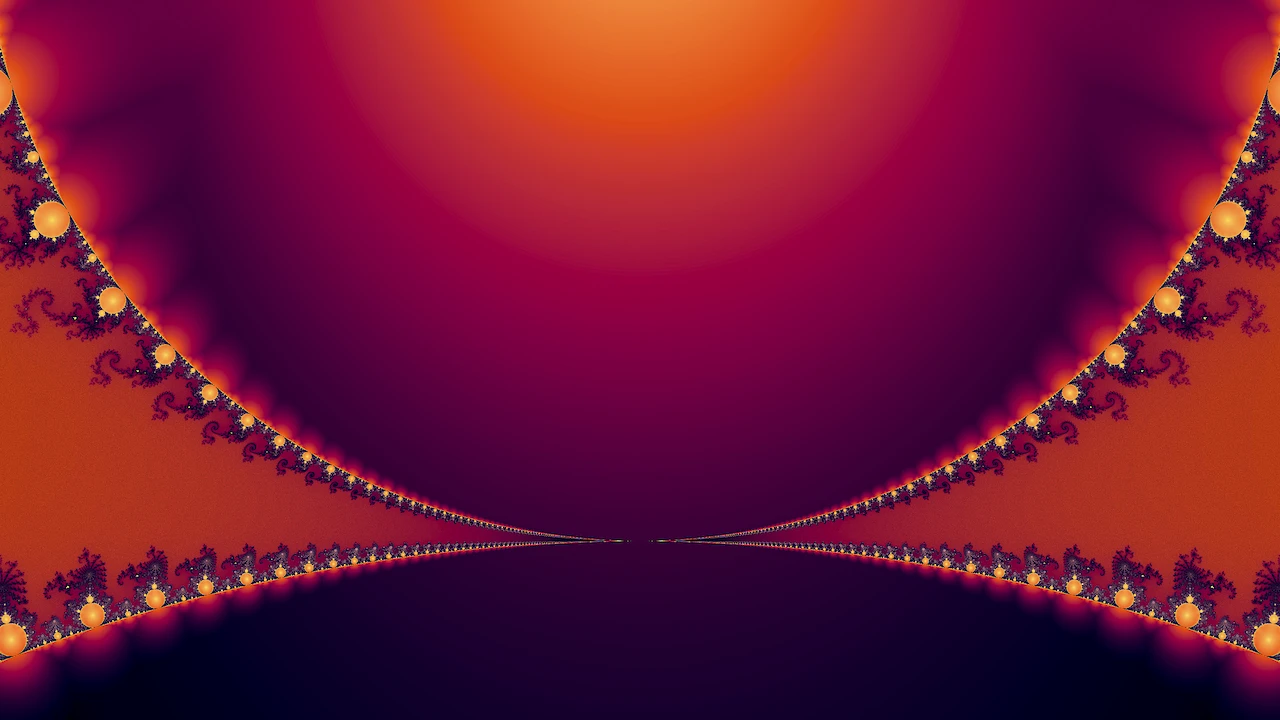
Return to Seahorse Valley
Mandelbrot
After a long break of doing mainly Buddhabrot renders I came to realize that my Mandelbrot collection was rather limited. When trying to explain some of my other images there was a growing need to have this one as a sort of reference.
The vanishing point where the two ridges of this image meet is the bifurcation point of the Mandelbrot set, at exactly c=-¾. The name 'Seahorse Valley' refers to the intricately detailed repeating structures that line the underside of the two fractal spikes. Above the seahorses a different 'double spiral' shape decorates the offspring of the period-2 bulb.
The inner part of the Mandelbrot set (which is usually colored black) is shown here with a coloring based on the orbit minimum distance from the origin. All orbits associated with the brightly colored inner regions very quickly converge to zero. And once a zₙ value becomes exactly zero the next z-value must be exactly z₁ (assuming we set z₀ equal to zero). From this point onwards the orbit repeats itself with perfect mathematical precision.
Although all orbits originating from within the Mandelbrot set are known to be converging, the dark branching structures reveal suspiciously organized variations in the speed at which this convergence occurs. Shortly after finishing this image I made a similar rendering of the Elephant Valley.